6 hours ago, Phoenix3 said:
1. Why? Why does it have to be changed? I know you say that one has to double the 2/3 subharmonic (which is yang) to create the 4/3 overtone (which is yin), and to do so, one has to change the direction so it can become an overtone, but why does doubling a ratio change the direction? Is it just a mathematical rule i’m ignorant about?
So this is the key secret of the Greek Miracle - the 9/8 as the major 2nd interval is based on "geometric magnitude" not based on frequency/wavelength. Remember that to subtract the Perfect Fifth from the PErfect Fourth the value is 9/8 but this has to only use 4/3 as the value of the PErfect Fourth and then ONLY 3/2 as the Perfect Fifth (not 2/3 even though 2/3 is also the PERfect fifth and the origin of the PErfect fourth). So the equation is Arithmetic Mean (3/2) x Harmonic mean (4/3) equals Geometric Mean SQuared (2). I will repost the quotes from the first page about this secret:
Professor Richard McKirahan, "On Philolaus and Number":
So instead of taking 12:9, which is 3/4 of 12, we take 8:6, which is 3/4 of 8. And so by adding the length 12 to 8 [as geometric magnitude not wavelength!!] with the length 8 to 6, [as geometric magnitude, not wavelength!!] we get the length 12 to 6, which corresponds to the ratio 2:1.
Professor Richard McKirahan reveals the secret:
The word translated epogdoic is not a musical term but a mathematical one. An epogdoic ratio is the ratio of 9 to 8. The occurrence of a mathematical term here is unexpected. It has been treated as an unimportant anomaly but in fact it is the key to the entire fragment....The word magnitude normally refers to physical size, but here it is given a new application, extending the notion of magnitude to include musical intervals.
These remarks raise the question of the difference between the ancient Pythagorean ‘musical’ perception as displayed in the Pythagorean idea of ‘linear number’ in Boethius [Philolaus] or in Nicomachus, and the modern ‘geometrical’ perception of the linear numerical magnitudes.
So everyone learns music theory as the Perfect Fifth PLUS the Perfect Fourth = the Octave. This is actually the first logarithm in Western science and it is ONLY possible by covering up the fact that 4/3 is derived from a different "root tonic" as the 1 - and then changing the direction of the "negative infinity" of 0. So the geometric magnitude is the the Perfect Fifth as 8/12 wavelength or 3/2 frequency (but again this is actually 3/2 as geometric magnitude now!) and then the PErfect Fourth is 8/6 as 4/3 frequency from 6/8 wavelength of 0 to 8 root tonic. And so by adding up those geometric magnitudes the answer is 2 as the Octave or 12 to 6 as 12 to 0 root tonic.
“Orthodox Pythagorean theory recognizes five consonances: fourth, fifth, octave, twelfth, and double octave; and these are represented by the multiple and superparticular ratios [n + 1 : n] from the tetrad. The number 8 obviously does not belong to the tetrad.”
André Barbera, "The Consonant Eleventh and the Expansion of the Musical Tetractys: A Study of Ancient Pythagoreanism," Journal of Music Theory, 1984, citing Ptolemy of 2nd century.
Professor Andrew Barker, Oxford, emeritus, p. 58, Scientific Method in Ptolemy's HarmonicsFrom the point of view of perception, as Ptolemy states (11.1-3) and as all parties to these enquiries agree, the fourth, fifth and octave are indubitably concordant, and this property belongs to no other interval within the octave.
2. I thought the octave is always 0 to 8 ( - C - D - E - F - G - A - B - C - ). I remember you said before that the old octave was 3 to 6, but I don’t know why the old octave was 3 to 6 (not just 0 to 6, or 0 to 8), and why it has to change from 3 to 6 to 0 to 8?
Yes the octave is half - or 1/2 the wavelength - so it is 0 to 8 and also 0 to 12 as the root tonics and so the 6 is the octave and 3 is also the octave but the 6 is as geometric magnitude as 12 to 0 in the opposite direction.
Math Professor Luigi Borzacchini argues that the Epinomis, authored by the Plato student Philippus of Opus, a member of the Academy, approximately contemporary of Aristotle and Eudoxus - this document is a "vanishing mediator" of the transfer from Pythagorean harmonics to the Greek Miracle of irrational magnitude:
Thus the first <analogy> [proportion] is of the double in point of number, passing from one to two in order of counting, and that which is according to power is double; that which passes to the solid and tangible is likewise again double, having proceeded from one to eight; but that of the double has a mean, as much more than the less as it is less than the greater, while its other mean exceeds and is exceeded by the same portion of the extremes themselves. Between six and twelve comes the whole-and-a-half (9=6+3) and whole-and-a-third (8=6+2):
3. I know that you said 2/3 of 1 (which is C to F) is 3, but I don’t understand why this is. To me, it is just 0.6666..., or 2/3(1). How does 0.6666... equal 3?
Decimal numbers already assume a real number value based on geometry.
The decimal system has been extended to infinite decimals, for representing any real number, by using an infinite sequence of digits after the decimal separator....
and
decimal number: a real number which expresses fractions on the base 10 standard numbering system using place value, e.g. 37⁄100 = 0.37
So again the concept of a real number is based on defining infinity as a visual process of measurement. Listening to the source of sound does not depend on vision! So listening is proven by science to be "faster than fourier uncertainty" or "time-frequency uncertainty" by 10 times. And so based on Hertz it is IMPOSSIBLE to listen to Perfect Intervals as integers - 2/3 and 3/2. But Daoist Harmonics is based on simple numbers, as is Pythagorean harmonics. So that is what we are talking about. If we want to use Hertz then we have to acknowledge that since the early 1900s - the foundation of science now is quantum mechanics and so time-frequency uncertainty which assumes symmetric math, is actually a "measurement problem" that is noncommutative - as an invariant observer ratio, as Alain Connes points out. So he calls it (2, 3, infinity) as the noncommutative phase mathematical structure that is the basis for the physics - and so the noncommutative structure is ALSO before decimals since they assume a symmetric "divide and average" math based on irrational geometry. So noncommutative phase is also BEFORE discrete numbers - so the "one" is a process of infinite listening as complementary opposites that are non-local as the 5th dimension.
Incommensurability, Music and Continuum: a cognitive approach. Arch. For History of Exact Sciences, 61 (2007) 273-302. http://dx.doi.org/10.1007/s00407-007-0125-0 https://www.academia.edu/16496242/Incommensurability_Music_and_Continuum_a_cognitive_approach._Arch._For_History_of_Exact_Sciences_61_2007_273-302._http_dx.doi.org_10.1007_s00407-007-0125-0
Our idea of (continuous) numerical magnitude is based on the idea of real number, a sequence of digits split by a decimal point:
... a3 a2 a1 . a-1 a-2 a-3 ....
It includes two infinite sharply different sequences: the left one is potentially infinite and in the realm of discreteness, the right one is actually infinite and in the realm of continuity.
Thus, continuous and discrete appear somehow intertwined: discrete magnitudes are special continuous magnitudes and a continuous magnitude can be represented by an infinite set of discrete magnitudes.
So as I quote Professor Borzacchini below - this does NOT apply to Pythagorean or Daoist Harmonics.
So the 3 as the frequency is based on the root tonic since the 2 is the SAME pitch as the 1. As I quoted - "time-frequency uncertainty" is due to defining frequency as a geometric cycle as 2pi/seconds. So frequency was actually only first created by Mersenne - after logarithms were invented. But quantum physics is the foundation of science now and so in fact frequency is based on "time-frequency uncertainty" - so as I quoted - the more decimal points you add the value of the frequency, the greater the precision of the frequency and so the less accurate the time value to measure the frequency. This is why frequency with no decimal points means a 2 Hertz inaccuracy for a 1 second cycle, as per time-frequency uncertainty. But this actually originates from noncommutative phase - before frequency was created.
So just as fractions are repeating decimals, irrational numbers are non-repeating decimals - but are defined officially as a "limit" as a process of the calculus. And so as Bertrand Russell stated "real numbers" are a "convenient fiction." The point being that arithmetic is based on distance while geometric is based on length. And so pure number is based on time as the 5th dimension that can not be seen. So to define number based on a geometric number line assumes the philosophical concept of defining infinity as a "contained" geometric continuum.
Math Professor Borzacchini:
And so pure number is based on time as the 5th dimension that can not be seen. As math professor Luigi Borzacchini states,As far as we know, in earlier Pythagoreanism the "linear numerical magnitude" was simply a line of indivisible monads and the difference between monad and point was only in "having position". This peculiar ‘perception’ of the points/numbers was clear in the abacus, and was linked to the already mentioned role of the One, which played in Greek philosophy a double role as both a quantity and a logical predicate. In the logical role any number was ‘one’ number and ‘one’ was indivisible and could not be a number. As unit of measurement ‘one’ was divisible but it had to change the ‘genus’, because it and its parts were heterogeneous, and in the original genus it was indivisible (Aristoteles 1831, Metaph. XIV.1). Even in modern non-decimal systems we have ‘foot’, ‘inch’, etc. i.e. heterogeneous units connected by a multiplicative factor.
The 'demusicalization' of the theory of proportions by Plato is shocking....If we consider the likely Pythagorean and Philolaic origin of the Timeus this 'removal' seems really astonishing!....Why these silences? And why this sudden and radical change? Why the Pythagoreans' silence? The "secret of the sect"? ....all the more because a purely negative result (speaking about "something which is not") had to fall under the blows of the negative judgement paradox. Such paradox forbade speaking about what is not....But a statement about what is not is about nothing and hence impossible....The refusal of speaking of "what is not" ...was the reason why musical incommensurability fell into oblivion....Continuum is not only inexpressible, but also external to the knowledge of reality....We can suppose that the Quadrivium in its earlier Pythagorean version did not know any discrete/continuous opposition. Math Professor Emeritus Luigi Borzacchini (origin of above 9th Century Boethius image from Philolaus (5th century BCE))
So we are talking about noncommutative phase as ratios - not decimals nor irrationals. So the Harmonic Series DIVERGES as fractions - it is defined by time, not by a geometric continuum.
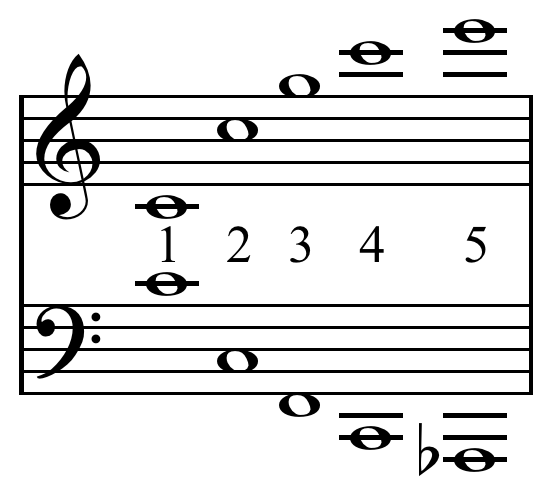
4. So the F is now the beginning of the new octave? Are you saying the scale is now F - G - A - B - C - D - E - F ? If F is the new root tonic, then why is it doubled to 4/3 as C to F? C to F is 2/3 with C as the root tonic, so 4/3 from F (which you say is the new root tonic) is B I think.
Nicolas Slonimsky once pointed out, in an effort to dissuade readers from the idea that Western tonality is the inevitable result of how we hear (as opposed to a largely artificial invention), that no matter how high one goes in the harmonic series, a fundamental pitch will not produce a perfect fourth above the fundamental.
So if we try to construct the scale from the Perfect Fifths - then we lose the 4/3 value of the F from the 2/3 subharmonic.
Dr. Oscar Abdounor:
And the PErfect Fourth of F is actually B flat - not B. But you got close.So what he doesn't realize - Dr. Oscar Abdounor - is that the issue is the noncommutative phase - this is why the Perfect Fifth and Perfect Fourth do not line up - even though the Perfect Fourth is constructed from the PErfect Fifth as an inversion.
The chromatic Pythagorean scale is:The notes F and c found in the table presented before, 131072:177147 = 0,73990527641 and 262144:531441 = 0,49327018427, respectively are slightly different from 3:4 and 1:2, which are those of the Pythagorean scale. as mentioned before, a do (c) was considered the initial note, so this result means that the fourth and the octave obtained by the aforementioned process are not
exactly the same obtained in the discovery with the monochord, attributed to Pythagoras, of the numerical ratios corresponding to the main intervals of the musical scale. One can put it down to the fact that the aforementioned cycles of the octaves and of fifths don’t meet, as it will be shown later and that the best approximation for this meeting occurs with 7 octaves and 12 fifths. So it is plausible that the ratios determined by the fourth and by the octave in the Pythagorean scale result from the experiment of the monochord, namely, from the musical perception that such ratios produce these intervals, they were the perfect consonances in the context of Greek music and which were capable of being produced by ratios composed by small numbers, being easier to relate to the intervals using only the ear....... Pythagorean tuning were first considered by musical theorists [445,7] in order to answer the need of a new musical language provided by polyphony. anachronistically speaking, this means that, supposing both these both cycles meet, there would be m and n integers such that (2:3)n = (1:2)m, that is, 3n = 2m+n, which is impossible, since the left term is odd and the right is even.

No comments:
Post a Comment