O.K. your question is precisely the secret of a great cover up that math professor Luigi Borzacchini published as "really astonishing" and "shocking" and created a "deep pre-established disharmony" that "guides the evolutive principle" of science.
So you just said "the circle of fifths" - but maybe you don't realize that the circle of fifths is a lie!! Yes I realize everyone learns the circle of fifths as basic music theory. I learned it in a music theory class in high school taught by my classical piano teacher.
So you have entered to the realm of why Western music theory and Western math is a lie - obviously that is a deep topic that most would never acknowledge that it exists. haha. I can go into that - but let's try to stay focused on Daoist music harmonics.
So first of all realize - o.k. hold on I'll get a graphic image for you.... to help explain...
O.K. so now you have officially entered into Western music theory - congratulations.
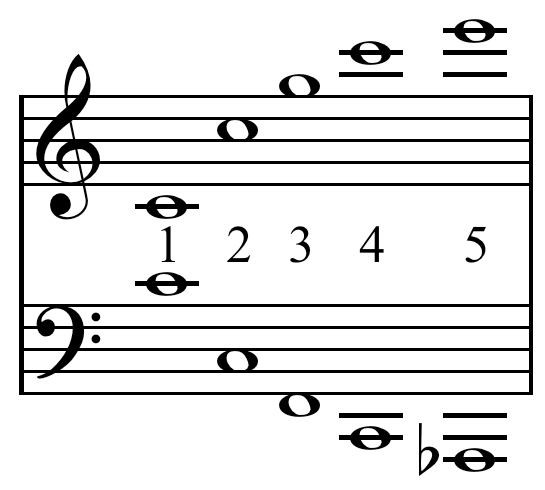
Notice first of all the "x" - what does the "x" refer to? It actually means - and they won't tell you this - but it means geometric magnitude as irrational geometry. In other words it means that sound as listening is inherently defined as a physical materialistic source - a vibrating string or one dimensional line.
Now notice that 2/3 - what you asked about - 2/3 is the subharmonic or from below - as the frequency and so the "x" is C as the root tonic while 2/3x is the Perfect Fifth as F to C, subharmonic. And so then 3/2x is the overtone harmonic as C to G.
O.K. so that's the "Doe a Deer" scale that everyone hears from the Sound of Music movie. But what people don't realize is that it originated from a lie! I can go into that in more detail.
But now that we have shown a concept visually - we can go back to the Daoist tuning.
So I call this the "bait and switch" - what happens is that when the music theory is taught - you have three factors - and one of them will get dropped. You have the geometry - so you have F to C as subharmonic and then C to G as overtone harmonic. Then you have the ratios or fractions. Notice that they are ratios with the "X" but the basic principle of Pythagoras is that frequency is inverse to wavelength and so they are fractions in that case. So you have 3/2 and 2/3. Then you have the third factor which is the pitch - and in relation to the fundamental pitch or the root tonic of C then both 3/2 and 2/3 are both the Perfect Fifth. And so that is why they are both Yang.
So your second question - why can't they just use the Perfect Fifth alone to create the scale, instead of using 4/3 and 3/2 - this is again the precise secret that no one notices. Well except math professor Alain Connes noticed it! So first of all we need to realize, as math professor Luigi Borzacchini did, that music theory created Western mathematics and not the other way around! This is a very powerful thing about music that hardly ever gets acknowledged.
O.K. so I have already mentioned that the circle of fifths is a lie which implies that the "spiral of fifths" is the truth. I have quoted Sir James Jeans, a quantum physicist, stating that indeed, in his book Science and Music, that the spiral of fifths, is the truth. Well it is not quite that simple, again due to the deeper math of music that Alain Connes noticed - and that the Daoists also knew. This is called "noncommutative phase" which means that G=3=F at the same time, as subharmonic and overtone and there is no fundamental pitch.
O.K. let's approach this from Western music theory again. There is a big cover up that is not talked about at all in Western music theory - it's the difference between the overtone series and the harmonic series. The basic point to understand is that the Perfect Fourth does not exist in the overtone series. I'll get you another graphic image....
O.K. so you can see now G=3=F at the same time.
But with the overtone series, the root tonic or fundamental pitch as 1 has to be the common harmonic for the multiple frequencies. So you have 3/2 as the Perfect Fifth because 2 is the same fundamental pitch as the 1 but you can not have 4/3 as the Perfect Fourth because 3 does not go into 2 evenly. So we learn that the Perfect Fifth x the Perfect Fourth equals the Octave but this is only the pitch and not the actual intervals. Again 2/3 is also the Perfect Fifth as the undertone.
What the "Doe a Deer" scale claims is that the Perfect Fifth minus the Perfect Fourth = 9/8 because to subtract then you multiply by the inverse. But again this ONLY works if you assume that the Perfect Fifth can only be 3/2 and not 2/3. So then you have 3/2 x 3/4=9/8 as the major 2nd music interval. So what we just covered up - without realizing it - is that 2/3 is also the Perfect Fifth as C to F undertone. And this is why there is an "x" in the music scale as the root tonic. This is also called the Phantom Tonic - because the Perfect Fourth as C to F or 4/3 actually changes the root tonic into 3 instead of 1 or 2 octave.
https://nmbx.newmusicusa.org/IV-The-Phantom-Tonic/
Please realize that what we are really talking about is "what is the one" - or what is the source of reality as time-consciousness! We can not see the source but we can listen to it.
And so in Daoist alchemy what we are doing is harmonizing what appears to be yin as 4/3 based on the appearance of symmetric form as beauty - back into the truth as formless time that is yang as 3/2 and 2/3, the Perfect Fifth. But this is an eternal process of energy-information as time-frequency and so it is never ending and never beginning. No "one" is listening - there is no "one" - and so the energy-information hears us and we listen to it and vice versa. It is the 5th dimension that is noncommutative phase at a zero point in space - there is 2/3 and 3/2 that is infinite since it is nonlocal as being in two places at the same time, faster than the speed of light.
So what I just described to you is hardly ever acknowledged in Western music theory! You can see it being acknowledged in Indian Rags music theory of the "three gunas" - https://books.google.com/books?id=hGLRqLscf78C&pg=PA72&lpg=PA72&dq=perfect+fourth+not+part+of+the+harmonic+series&source=bl&ots=gIduz2q6SS&sig=KecQSitfXhPDoF_mBwPuv6Z5YxA&hl=en&sa=X&ved=0ahUKEwjCkrOunOLZAhUHKGMKHRGpA8c4ChDoAQhMMAY#v=onepage&q=perfect fourth not part of the harmonic series&f=false
Why? Because ever since Philolaus and Archytas - and Plato - we actually define the fundamental pitch as geometric magnitude that is irrational - and so for Philolaus the "x" was actually the 9/8 as the major 2nd note and it was derived by assuming the octave as 2 is the geometric mean squared (or "x squared" = 2) so that 3/2 x 4/3 = 2 while also the Perfect Fifth plus the Perfect Fourth = the Octave. So again we think this is basic music theory but it is actually a lie! Why? Because the root tonic has to be changed in order to make this work - and then it is claimed the root tonic is this contained infinity as the geometric mean, so therefore each note as 9/8 has to be "compromised" to line up the 3 and 2 or the Perfect Fifth into the same octave.
So you ask why do the Daoists change from 3/2 and 4/3 - and so this brings us to another deep mystery of listening versus the visual measurement of music based on frequency and wavelength. In other words again we have three factors - pitch and then the ratios as geometry and then the numbers.
So this is the harmonic series as based on Fourier analysis of amplitude and frequency. So if you shift ...
O.K. so what this means is that we actually can hear sound faster than time-frequency uncertainty or the visual measurement of sound.
So this whole mathematical problem of trying to line up 2 and 3 - stating the numbers do not line up in time as a visual measurement of octave and perfect fifth - it does not exist for the EAR - the Yang and Yin harmonize as undivided Yuan Qi at the same time - and faster than the speed of light - as the 5th dimension of the future and past together.
In science this is called the "measuring problem" of quantum physics. Again it originates from music theory! But of course science does not normally recognize this. haha.
O.K. so Western science is now founded on quantum physics which in turn is founded on "time-frequency uncertainty" as the "measurement problem." This means at time zero there is nonlocal quantum entanglement or quantum nonlocality. There is a big debate about what this means. But the problem about this debate is that quantum physics is closely tied in with relativity and so actually only if relativity is also considered then does this problem get solved. And this is what de Broglie did with his "Law of Phase Harmony."
But let's get back to Daoist music tuning - you ask - why do they use 4/3 and not just the Perfect Fifth.... we have to delve into the math again. If you use just the Perfect Fifths then you do not actually get a Perfect Fourth as 4/3 in the cycle of fifths. Think of 3/4 as .75 and the octave 1/2 as .5 but with the cycle of fifths instead you get F to C as .739 and so it seems close but what is happening is that the difference is being resonated as a larger error. As I cited Sir James Jeans above - the difference in tuning when you go back to the octave based on the cycle of fifths is actually a quarter tone of the interval. And so as Sir James Jeans acknowledges - the truth if the infinite spiral of fifths since 2 and 3 do not line up.
But it's not quite that simple - what the ancients realized is that since 8 and 9 are successive integers then if the major 2nd interval is 9/8 from the Perfect Fifth minus the Perfect Fourth or 3/2 x 3/4 then to subdivide 9/8 evenly or the ratio of 8:9 - you need 8 to x as x is to 9 as a continued proportion, thereby proving that it can not be subdivided with an integer ratio. Another way to state this is that 9/8 as the major 2nd note with 6 of them is greater than the octave. And so when we learn the Pythagorean Comma it is supposedly the difference between the Octave and the Perfect Fifth cycle but in fact it is already assuming this 9/8 value with the octave defined as that which can be evenly divided into itself.
So you can see here the math is quite subtle. To state that 3/2 as the Perfect Fifth does not equal the octave is not the same as assuming that the 2 of the 3/2 can be evenly divided into the 3 and therefore that 3 multiplied by itself does not line up with 2 multiplied by itself. Why? Because 3/2 is also 2/3 as the Perfect Fifth!! Alain Connes realized this was the truth but most mathematicians cover this up. Sir James Jeans defined the Pythagorean Comma as based on 3/2 but most mathematicians assume that just 3 can be used - and so the noncommutative pitch of 3/2 is lost - in relation to the root tonic. In other words if you have C as the root tonic then 3 to 2 is different as G to C, the Perfect Fourth with C as the Octave as 4. So 3/4 is G to C while 4/3 is C to F. It is noncommutative phase in relation to the root tonic.
O.k. so I'll give you an example of how a math professor will do the "bait and switch":
The emergence of the idea of irrationality in Renaissance theoretical music contexts pdf
So the problem is that he doesn't realize that the irrationality was already discovered or promoted by Plato, Archytas and Philolaus.So here is what Alain Connes states:
- Alain Connes
Now notice he is using 3 to the 19th power and 2 to the 12th power but as Sir James Jeans defined the Pythagorean Comma of the difference between the spiral of fifths and octaves is (3/2) to the 12th and 2 to the 7th power. So what most mathematicians do is just "add" the multiplication as a logarithm with the assumption that (3/2) is the same as 3 multiplied by itself - but it is NOT because 2/3 is also the Perfect Fifth! Alain Connes realizes this secret when he says they are noncommutative.
What Dr. Abdounur states it is impossible to add these logarithms since the "left term" is "odd" - meaning 3 and the right term is even - meaning 2. So to say that (3:2) as a ratio to the 12th compared to 2 to the 7th with (1:2) as a ratio does not equal 3 to the 12th as 2 to the (7th plus 12th) because 3 is odd and 2 is even.
But what is really going on here is that the 1 or the one as the root tonic or fundamental pitch got changed in value!
In other words Western science assumes you have to start with a physical materialistic ratio as a geometric medium - a visual definition of time. But in Daoist and Indian harmonics - as a science of alchemy - this is not true! Instead we listen to time.
So again only Alain Connes has realized the empirical truth of the music tuning. He has a lecture on the Quantum Music of the Spheres:
O.K. so now we can go back to quantum physics....
You having fun yet? Because time was originally defined in the West as geometric magnitude then it takes a certain amount of time to make the measurement - inherent to the spatial change.
A quantum physicist gives an excellent music theory explanation of infinity from the time-frequency uncertainty principle:
O.K. this is NOT true for actual human hearing as it has been proven that human hearing or listening is faster than Fourier Uncertainty. And listening is an active process - so by training as a musician you actually rewire your brain. It's been proven that by training as a musician intensively from before 7 years old then you have a much larger corpus callosum. No other Western type of training does that.So the interference beat is the subharmonic and so based on this concept of frequency as inverse to time - there is an inherent uncertainty in the measurement that can not be precise, based on a visual measurement of time and frequency. This even occurs at the speed of light since light is a visual measurement. But when we listen we can bypass this inherent uncertainty.
So time-frequency uncertainty means that as you go to a smaller pulse of time that hears the sound then the frequency gets higher but in relation to the fundamental pitch or root tonic the difference increases in hertz frequency you hear less of a difference in the frequencies and so need less time to notice the difference. And so in music theory this is called the "paradox of the poles."
This is how Sir James Jeans explained it - as I quote in my master's thesis.
and
Jeans provides the following on the topic:
But what is found is that in actual human listening - as the beats disappear from time-frequency uncertainty - and we can hear faster than fourier uncertainty - so we don't define the octave as a circle on the oscilloscope - but rather we define the octave as Sir James Jeans defined it - that there are so many subharmonic beats at the octave of the fundamental root tonic that the beats disappear! And when this is tested based on Fourier Uncertainty - what was discovered is that as the frequency goes higher and higher and so the time of the sound is less and less as just a click sound - the fundamental pitch then shifts up a Perfect Fifth in frequency.
So
Emilio Del Guidice
we are talking about a noncommutative phase shift that is faster than Fourier Uncertainty which is the "measurement problem" in quantum physics at the speed of light of relativity - that hides quantum nonlocality.
Listening is 10 times faster than Fourier Uncertainty! Planck's Constant is an "action" with the time variable hidden within it - as cycles (wavelength defined by commutative PI phase) per second. In fact Planck's Constant is just the mean energy of light in one cycle of time - just by dividing frequency of light by cycles per second you get Planck's constant.
To quote Alain Connes again:
So you can watch his Quantum music of the spheres lecture - where he creates music frequencies based on this concept of the triple spectal as noncommutative phase. But we are talking about a unified field theory of science from music theory! haha.
The ancients already understood this based on quantum biology as listening as meditation.
“Human hearing beats the Fourier uncertainty principle” Feb 04, 2013 by Lisa Zyga link here

No comments:
Post a Comment